Linear equations

Got it! This site "robinsnyder.com" uses cookies. You consent to this by clicking on "Got it!" or by continuing to use this website. Note: This appears on each machine/browser from which this site is accessed.

There are many ways to represent dimensions.

Geometry is used here for a discussion on dimensions.

Any two distinct points in a plane determine a line in the plane.

A line can depict one dimension, called 1D.

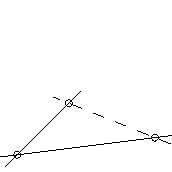
Three distinct points determine a plane.

A plane can depict two dimensions, called 2D.

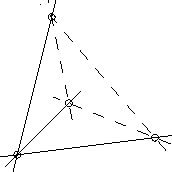
Four distinct points determine a space.

A space can depict three dimensions, called 3D.

Five or more distinct points determine a hyper-space.

A hyper-space can depict four or more dimensions, called 4D (or more).

Since humans have trouble visualizing even four dimensions, the term hype-space will be used for 4D space.

Unless otherwise specified, hyper-spaces will refer to 4 dimensions.

The mathematician Hilbert generalized dimensions to a potentially infinite number of dimensions, called Hilbert spaces.

The purpose of visualization of concepts is often go get in intuitive feel/view for what is happening.

The results and methods are then generalized to many more dimensions.

-

A line in two dimensions has the following form.

y = f(x) = m * x + b

The following hold.

The variable y is the dependent variable (i.e., y is a function of x). and
The variable x is the independent variable (i.e., x functionally determines y).
The value m is the slope if the line.
The value b is the intercept of the line on the y axis (i.e., when x is 0).

What is a dependent variable? Give a specific example.

What is an independent variable? Give a specific example.

The slope is defined as the rise over the run.

In the linear equation

y = m * x + b

m is the slope.

The slope is the rise over the run.

The run is the horizontal displacement.
The rise is the vertical displacement.

For any two points x₁ and x₂ on the line, the run, or horizontal displacement, is

x2 - x1.

The corresponding rise, or vertical displacement, is

f(x2) - f(x1) = y2 - y1

Substituting the definition of f(x) in the rise yields the following.

f(x2) - f(x1) = y2 - y1 = (m * x2 + b) - (m * x1 + b) = m (x2 - x1)

So, the slope m is the following.

rise / run = m * (x2 - x1) / (x2 - x1) = m

The slope can also be defined as the change in f(x) per unit change in x.

slope = f(x+1) - f(x) = m*(x+1)+b - (m*x+b) = m*x+m+b -m*x-b = m

In math, an intercept is the point at which two geometric objects cross paths. In football, a defensive player can intercept a pass thrown by an offensive player.

In a line, the y-intercept is the value of y when the line intersects the x-axis (i.e., when x is 0). So, the y-intercept is

f(0) = m * 0 + b = b.

So, the slope is m and the intercept is b.

To graph a line, two points are needed. In general, it is best to pick the two points that are easiest to graph.

Consider the following points.

(x1, y1) = (5, 7) (x2, y2) = (15, 17)

Since these are not the same points, these points define a line.

(x1, y1) = (5, 7) (x2, y2) = (15, 17)

What is the horizontal displacement?

The horizontal displacement is x2-x1 = 15-5 = 10.

What is the vertical displacement?

The vertical displacement is y2-y1 = f(x2)-f(x1) = 17-7 = 10.

(x1, y1) = (5, 7) (x2, y2) = (15, 17)

What is the slope of the line?

The slope of the line is determined as follows.

slope = m = rise / run = (17-7) / (15 - 5) = 10 / 10 = 1

(x1, y1) = (5, 7) (x2, y2) = (15, 17)

What is the y-intercept of the line? Since

y = m * x + b

we know the following, where m is 1.

y2 = m * x2 + b y1 = m * x1 + b

Thus we have the following two equations.

17 = 15 + b 7 = 5 + b

Solving either one will yield the result that the y-intercept b = 2.